General
- Details
- Parent Category: Math League Website
What is an angle? Two rays that share the same endpoint form an angle. The point where the rays intersect is called the vertex of the angle. The two rays are called the sides of the angle. Example: Here are some examples of angles.Angles and angle terms
Degrees: measuring angles
Acute angles
Obtuse angles
Right angles
Complementary angles
Supplementary angles
Vertical angles
Alternate interior angles
Alternate exterior angles
Corresponding angles
Angle bisector
Perpendicular lines
What is an Angle?
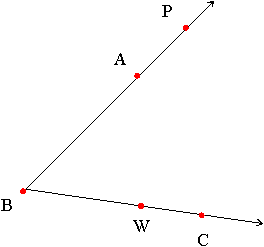
We can specify an angle by using a point on each ray and the vertex. The angle below may be specified as angle ABC or as angle CBA; you may also see this written as Example: Many different names exist for the same angle. For the angle below, 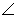 ABC or as
ABC or as CBA. Note how the vertex point is always given in the middle.
CBA. Note how the vertex point is always given in the middle.
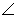 PBC,
PBC,  PBW,
PBW,  CBP, and
CBP, and  WBA are all names for the same angle.
WBA are all names for the same angle.
We measure the size of an angle using degrees. Example: Here are some examples of angles and their degree measurements.
Degrees: Measuring Angles
An acute angle is an angle measuring between 0 and 90 degrees. Example: The following angles are all acute angles.
Acute Angles
An obtuse angle is an angle measuring between 90 and 180 degrees. Example: The following angles are all obtuse.
Obtuse Angles
A right angle is an angle measuring 90 degrees. Two lines or line segments that meet at a right angle are said to be perpendicular. Note that any two right angles are supplementary angles (a right angle is its own angle supplement). Example: The following angles are both right angles.
Right Angles
Two angles are called complementary angles if the sum of their degree measurements equals 90 degrees. One of the complementary angles is said to be the complement of the other. Example: These two angles are complementary.
Complementary Angles
Note that these two angles can be "pasted" together to form a right angle!
Two angles are called supplementary angles if the sum of their degree measurements equals 180 degrees. One of the supplementary angles is said to be the supplement of the other. Example: These two angles are supplementary.
Supplementary Angles
Note that these two angles can be "pasted" together to form a straight line!
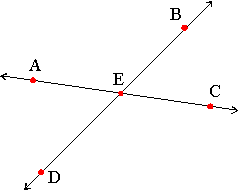
For any two lines that meet, such as in the diagram below, angle AEB and angle DEC are called vertical angles. Vertical angles have the same degree measurement. Angle BEC and angle AED are also vertical angles.
Vertical Angles
For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle D are called alternate interior angles. Alternate interior angles have the same degree measurement. Angle B and angle C are also alternate interior angles.
Alternate Interior Angles
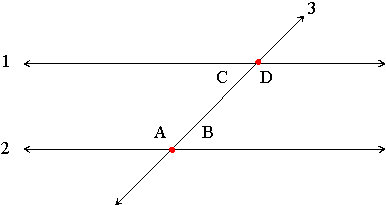
For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle D are called alternate exterior angles. Alternate exterior angles have the same degree measurement. Angle B and angle C are also alternate exterior angles.
Alternate Exterior Angles

For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle C are called corresponding angles. Corresponding angles have the same degree measurement. Angle B and angle D are also corresponding angles.
Corresponding Angles

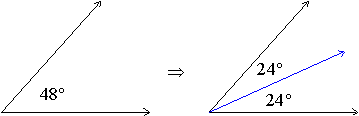
An angle bisector is a ray that divides an angle into two equal angles. Example:
Angle Bisector
The blue ray on the right is the angle bisector of the angle on the left.
The red ray on the right is the angle bisector of the angle on the left.
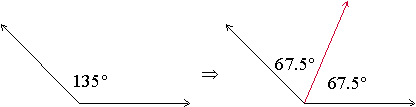
Two lines that meet at a right angle are perpendicular.
Perpendicular Lines
- Details
- Parent Category: Math League Website
Lines A line is one of the basic terms in geometry. We may think of a line as a "straight" line that we might draw with a ruler on a piece of paper, except that in geometry, a line extends forever in both directions. We write the name of a line passing through two different points A and B as "line AB" or as Example: The following is a diagram of two lines: line AB and line HG.Basic terms
Points
Intersection
Line segments
Rays
Endpoints
Parallel lines
Lines
, the two-headed arrow over AB signifying a line passing through points A and B.
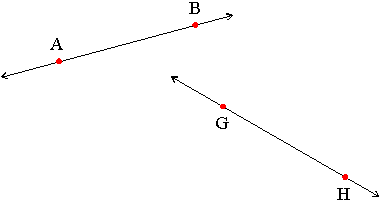
The arrows signify that the lines drawn extend indefinitely in each direction. A point is one of the basic terms in geometry. We may think of a point as a "dot" on a piece of paper. We identify this point with a number or letter. A point has no length or width, it just specifies an exact location. Example: The following is a diagram of points A, B, C, and Q:
Points

The term intersect is used when lines, rays, line segments or figures meet, that is, they share a common point. The point they share is called the point of intersection. We say that these figures intersect. Example: In the diagram below, line AB and line GH intersect at point D:
Intersection

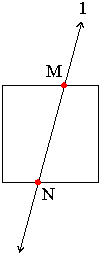
Example: In the diagram below, line 1 intersects the square in points M and N:
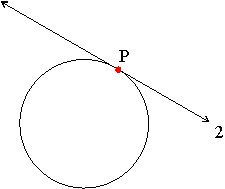
Example: In the diagram below, line 2 intersects the circle at point P:
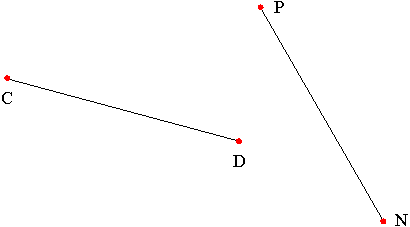
A line segment is one of the basic terms in geometry. We may think of a line segment as a "straight" line that we might draw with a ruler on a piece of paper. A line segment does not extend forever, but has two distinct endpoints. We write the name of a line segment with endpoints A and B as "line segment AB" or as Example: The following is a diagram of two line segments: line segment CD and line segment PN, or simply segment CD and segment PN.
Line Segments
. Note how there are no arrow heads on the line over AB such as when we denote a line or a ray.
A ray is one of the basic terms in geometry. We may think of a ray as a "straight" line that begins at a certain point and extends forever in one direction. The point where the ray begins is known as its endpoint. We write the name of a ray with endpoint A and passing through a point B as "ray AB" or as Example: The following is a diagram of two rays: ray HG and ray AB.
Rays
. Note how the arrow heads denotes the direction the ray extends in: there is no arrow head over the endpoint.

An endpoint is a point used to define a line segment or ray. A line segment has two endpoints; a ray has one. Example: The endpoints of line segment DC below are points D and C, and the endpoint of ray MN is point M below:
Endpoints
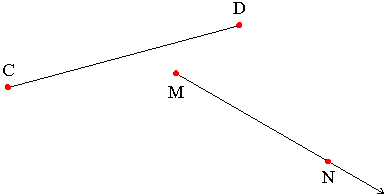
Two lines in the same plane which never intersect are called parallel lines. We say that two line segments are parallel if the lines that they lie on are parallel. If line 1 is parallel to line 2, we write this as line 1 || line 2 When two line segments DC and AB lie on parallel lines, we write this as segment DC || segment AB. Example: Lines 1 and 2 below are parallel.
Parallel Lines
Example: The opposite sides of the rectangle below are parallel. The lines passing through them never meet.
- Details
- Parent Category: Math League Website
Prime numbers A whole number greater than one that is divisible by only 1 and itself. The numbers 2, 3, 5, 37, and 101 are some examples of prime numbers. The greatest common factor of two or more whole numbers is the largest whole number that divides each of the numbers. There are two methods of finding the greatest common factor of two numbers. Method 1: List all the factors of each number, then list the common factors and choose the largest one. Example: 36: 1, 2, 3, 4, 6, 9, 12, 18, 36 54: 1, 2, 3, 6, 9, 18, 27, 54 The common factors are: 1, 2, 3, 6, 9, and 18. The greatest common factor is: 18. Method 2: List the prime factors, then multiply the common prime factors. Example: 36 = 2 × 2 × 3 × 3 54 = 2 × 3 × 3 × 3 The common prime factors are 2, 3, and 3. The greatest common factor is 2 × 3 × 3 = 18.. The least common multiple of two or more nonzero whole numbers is the smallest whole number that is divisible by each of the numbers. There are two common methods for finding the least common multiple of 2 numbers. Method 1: List the multiples of each number, and look for the smallest number that appears in each list. Example: Find the least common multiple of 12 and 42. We list the multiples of each number: 12: 12, 24, 36, 48, 60, 72, 84, ... 42: 42, 84, 126, 168, 190, ... We see that the number 84 is the smallest number that appears in each list. Method 2: Factor each of the numbers into primes. For each different prime number in either of the factorizations, follow these steps: 1. Count the number of times it appears in each of the factorizations. 2. Take the largest of these two counts. 3. Write down that prime number as many times as the count in step 2. To find the least common multiple take the product of all of the prime numbers written down in steps 1, 2, and 3. Example: Find the least common multiple of 24 and 90. First, we find the prime factorization of each number. 24 = 2 × 2 × 2 × 3 90 = 2 × 3 × 3 × 5 The prime numbers 2, 3, and 5 appear in the factorizations. We follow steps 1 through 3 for each of these primes. The number 2 occurs 3 times in the first factorization and 1 time in the second, so we will use three 2's. The number 3 occurs 1 time in the first factorization and 2 times in the second, so we will use two 3's. The number 5 occurs 0 times in the first factorization and 1 time in the second factorization, so we will use one 5. The least common multiple is the product of three 2's, two 3's, and one 5. 2 × 2 × 2 × 3 × 3 × 5 = 360 Example: Find the least common multiple of 14 and 49. First, we find the prime factorization of each number. 14 = 2 × 7 49 = 7 × 7 The prime numbers 2 and 7 appear in the factorizations. We follow steps 1 through 3 for each of these primes. The number 2 occurs 1 times in the first factorization and 0 times in the second, so we will use one 2. The number 7 occurs 1 time in the first factorization and 2 times in the second, so we will use two 7's. The least common multiple is the product of one 2 and two 7's. 2 × 7 × 7 = 98 Examples: Some other least common multiples are listed below. The least common multiple of 12 and 9 is 36. The least common multiple of 6 and 18 is 18. The least common multiple of 2, 3, 4, and 5 is 60. A fraction is a number that expresses part of a group. Fractions are written in the form The number a is called the numerator, and the number b is called the denominator. Examples: The following numbers are all fractions Example: The fraction 4/6 represents the shaded portion of the circle below. There are 6 pieces in the group, and 4 of them are shaded.Fractions
Greatest common factor
Least common multiple
What is a fraction?
Equivalent fractions
Comparing fractions
Converting and reducing fractions
Lowest terms
Improper fractions
Mixed numbers
Converting mixed numbers to improper fractions
Converting improper fractions to mixed numbers
Writing a fraction as a decimal
Rounding a fraction to the nearest hundredth
Adding and subtracting fractions
Adding and subtracting mixed numbers
Multiplying fractions and whole numbers
Multiplying fractions and fractions
Multiplying mixed numbers
Reciprocal
Dividing fractions
Dividing mixed numbers
Simplifying complex fractions
Repeating decimals
Prime Numbers
Greatest Common Factor
Least Common Multiple
What is a Fraction?
or a/b, where a and b are whole numbers, and the number b is not 0. For the purposes of these web pages, we will denote fractions using the notation a/b, though the preferred notation is generally
.
1/2, 3/7, 6/10, 4/99

Example: The fraction 3/8 represents the shaded portion of the circle below. There are 8 pieces in the group, and 3 of them are shaded.
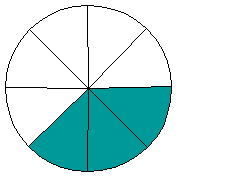
Example: The fraction 2/3 represents the shaded portion of the circle below. There are 3 pieces in the group, and 2 of them are shaded.

Equivalent fractions are different fractions which name the same amount. Examples: The fractions 1/2, 2/4, 3/6, 100/200, and 521/1042 are all equivalent fractions. Example: Test if 3/7 and 18/42 are equivalent fractions. Example: Test if 2/4 and 13/20 are equivalent fractions. 1. To compare fractions with the same denominator, look at their numerators. The larger fraction is the one with the larger numerator. Example: Compare the fractions 3/7 and 1/2. Example: Compare the fractions 13/20 and 3/5. For any fraction, multiplying the numerator and denominator by the same nonzero number gives an equivalent fraction. We can convert one fraction to an equivalent fraction by using this method. Examples: 1/2 = (1 × 3)/(2 × 3) = 3/6 2/3 = (2 × 2)/(3 × 2) = 4/6 3/5 = (3 × 4)/(5 × 4) = 12/20 Another method of converting one fraction to an equivalent fraction is by dividing the numerator and denominator by a common factor of the numerator and denominator. Examples: 20/42 = (20 ÷ 2)/(42 ÷ 2) = 10/21 36/72 = (36 ÷ 3)/(72 ÷ 3) = 12/24 9/27 = (9 ÷ 3)/(27 ÷ 3) = 3/9 When we divide the numerator and denominator of a fraction by their greatest common factor, the resulting fraction is an equivalent fraction in lowest terms. A fraction is in lowest terms when the greatest common factor of its numerator and denominator is 1. There are two methods of reducing a fraction to lowest terms. Method 1: Divide the numerator and denominator by their greatest common factor. 12/30 = (12 ÷ 6)/(30 ÷ 6) = 2/5 Method 2: Divide the numerator and denominator by any common factor. Keep dividing until there are no more common factors. 12/30 = (12 ÷ 2)/(30 ÷ 2) = 6/15 = (6 ÷ 3)/(15 ÷ 3) = 2/5 Improper fractions have numerators that are larger than or equal to their denominators. Examples: 11/4, 5/5, and 13/2 are improper fractions. Mixed numbers have a whole number part and a fraction part. Examples: To change a mixed number into an improper fraction, multiply the whole number by the denominator and add it to the numerator of the fractional part. Examples: 2 3/4 = ((2 × 4) + 3)/4 =11/4 6 1/2 = ((6 × 2) + 1)/2 = 13/2 To change an improper fraction into a mixed number, divide the numerator by the denominator. The remainder is the numerator of the fractional part. Examples: 11/4 = 11 ÷ 4 = 2 r3 = 2 3/4 13/2 = 13 ÷ 2 = 6 r1 = 6 1/2 Method 1 - Convert to an equivalent fraction whose denominator is a power of 10, such as 10, 100, 1000, 10000, and so on, then write in decimal form. Examples: 1/4 = (1 × 25)/(4 × 25) = 25/100 = 0.25 3/20 = (3 × 5)/(20 × 5) = 15/100 = 0.15 9/8 = (9 × 125)/(8 × 125) = 1125/1000 = 1.125 Method 2 - Divide the numerator by the denominator. Round to the decimal place asked for, if necessary. Example: 13/4 = 13 ÷ 4 = 3.25 Example: Convert 3/7 to a decimal. Round to the nearest thousandth. We divide one decimal place past the place we need to round to, then round the result. 3/7 = 3 ÷ 7 = 0.4285… which equals 0.429 when rounded to the nearest thousandth. Example: Convert 4/9 to a decimal. Round to the nearest hundredth. We divide one decimal place past the place we need to round to, then round the result. 4/9 = 4 ÷ 9 = 0.4444… which equals 0.44 when rounded to the nearest hundredth. Divide to the thousandths place. If the last digit is less than 5, drop it. This is particularly useful for converting a fraction to a percent, if we want to convert to the nearest percent. 1/3 = 1 ÷ 3 = 0.333… which rounds to 0.33 If the last digit is 5 or greater, drop it and round up. 2/7 = 2 ÷ 7 = 0.285 which rounds to 0.29 If the fractions have the same denominator, their sum is the sum of the numerators over the denominator. If the fractions have the same denominator, their difference is the difference of the numerators over the denominator. We do not add or subtract the denominators! Reduce if necessary. Examples: 3/8 + 2/8 = 5/8 9/2 - 5/2 = 4/2 = 2 If the fractions have different denominators: Example: 3/4 + 1/6 = ? The least common denominator is 12. 3/4 + 1/6 = 9/12 + 2/12 = 11/12. Example: 9/10 - 1/2 = ? The least common denominator is 10. 9/10 - 1/2 = 9/10 - 5/10 = 4/10 = 2/5. Example: 2/3 + 2/7 = ? The least common denominator is 21 2/3 + 2/7 = 14/21 + 6/21 = 20/21. To add or subtract mixed numbers, simply convert the mixed numbers into improper fractions, then add or subtract them as fractions. Example: 9 1/2 + 5 3/4 = ? Converting each number to an improper fraction, we have 9 1/2 = 19/2 and 5 3/4 = 23/4. We want to calculate 19/2 + 23/4. The LCM of 2 and 4 is 4, so 19/2 + 23/4 = 38/4 + 23/4 = (38 + 23)/4 = 61/4. Converting back to a mixed number, we have 61/4 = 15 1/4. The strategy of converting numbers into fractions when adding or subtracting is often useful, even in situations where one of the numbers is whole or a fraction. Example: 13 - 1 1/3 = ? In this situation, we may regard 13 as a mixed number without a fractional part. To convert it into a fraction, we look at the denominator of the fraction 4/3, which is 1 1/3 expressed as an improper fraction. The denominator is 3, and 13 = 39/3. So 13 - 1 1/3 = 39/3 - 4/3 = (39-4)/3 = 35/3, and 35/3 = 11 2/3. Example: 5 1/8 - 2/3 = ? This time, we may regard 2/3 as a mixed number with 0 as its whole part. Converting the first mixed number to an improper fraction, we have 5 1/8 = 41/8. The problem becomes 5 1/8 - 2/3 = 41/8 - 2/3 = 123/24 - 16/24 = (123 - 16)/24 = 107/24. Converting back to a mixed number, we have 107/24 = 4 11/24. Example: 92 + 4/5 = ? This is easy. To express this as a mixed number, just put the whole number and the fraction side by side. The answer is 92 4/5. To multiply a fraction by a whole number, write the whole number as an improper fraction with a denominator of 1, then multiply as fractions. Example: 8 × 5/21 = ? We can write the number 8 as 8/1. Now we multiply the fractions. 8 × 5/21 = 8/1 × 5/21 = (8 × 5)/(1 × 21) = 40/21 Example: 2/15 × 10 = ? We can write the number 10 as 10/1. Now we multiply the fractions. 2/15 × 10 = 2/15 × 10/1 = (2 × 10)/(15 × 1) = 20/15 = 4/3 When two fractions are multiplied, the result is a fraction with a numerator that is the product of the fractions' numerators and a denominator that is the product of the fractions' denominators. Example: 4/7 × 5/11 = ? The numerator will be the product of the numerators: 4 × 5, and the denominator will be the product of the denominators: 7 × 11. The answer is (4 × 5)/(7 × 11) = 20/77. Remember that like numbers in the numerator and denominator cancel out. Example: 14/15 × 15/17 = ? Since the 15's in the numerator and denominator cancel, the answer is 14/15 × 15/17 = 14/1 × 1/17 = (14 × 1)/(1 × 17) = 14/17 Example: 4/11 × 22/36 = ? In the solution below, first we cancel the common factor of 11 in the top and bottom of the product, then we cancel the common factor of 4 in the top and bottom of the product. 4/11 × 22/36 = 4/1 × 2/36 = 1/1 × 2/9 = 2/9 To multiply mixed numbers, convert them to improper fractions and multiply. Example: 4 1/5 × 2 2/3 = ?. Converting to improper fractions, we get 4 1/5 = 21/5 and 2 2/3 = 8/3. So the answer is 4 1/5 × 2 2/3 = 21/5 × 8/3 = (21 × 8)/(5 × 3) = 168/15 = 11 3/15. Examples: 3/4 × 1 1/8 = 3/4 × 9/8 = 27/32. 3 × 7 3/4 = 3 × 31/4 = (3 × 31)/4 = 93/4 = 23 1/4. The reciprocal of a fraction is obtained by switching its numerator and denominator. To find the reciprocal of a mixed number, first convert the mixed number to an improper fraction, then switch the numerator and denominator of the improper fraction. Notice that when you multiply a fraction and its reciprocal, the product is always 1. Example: Find the reciprocal of 31/75. We switch the numerator and denominator to find the reciprocal: 75/31. Example: Find the reciprocal of 12 1/2. First, convert the mixed number to an improper fraction: 12 1/2 = 25/2. Next, we switch the numerator and denominator to find the reciprocal: 2/25. To divide a number by a fraction, multiply the number by the reciprocal of the fraction. Examples: 7 ÷ 1/5 = 7 × 5/1 = 7 × 5 = 35 1/5 ÷ 16 = 1/5 ÷ 16/1 = 1/5 × 1/16 = (1 × 1)/(5 × 16) = 1/80 3/5 ÷ 7/12 = 3/5 × 12/7 = (3 × 12)/(5 × 7) = 36/35 or 1 1/35
Equivalent Fractions
The fractions 3/7, 6/14, and 24/56 are all equivalent fractions.
We can test if two fractions are equivalent by cross-multiplying their numerators and denominators. This is also called taking the cross-product.
The first cross-product is the product of the first numerator and the second denominator: 3 × 42 = 126.
The second cross-product is the product of the second numerator and the first denominator: 18 × 7 = 126.
Since the cross-products are the same, the fractions are equivalent.
The first cross-product is the product of the first numerator and the second denominator: 2 × 20 = 40.
The second cross-product is the product of the second numerator and the first denominator: 4 × 13 = 52.
Since the cross-products are different, the fractions are not equivalent. Since the second cross-product is larger than the first, the second fraction is larger than the first.
Comparing Fractions
2. To compare fractions with different denominators, take the cross product. The first cross-product is the product of the first numerator and the second denominator. The second cross-product is the product of the second numerator and the first denominator. Compare the cross products using the following rules:
a. If the cross-products are equal, the fractions are equivalent.
b. If the first cross product is larger, the first fraction is larger.
c. If the second cross product is larger, the second fraction is larger.
The first cross-product is the product of the first numerator and the second denominator: 3 × 2 = 6.
The second cross-product is the product of the second numerator and the first denominator: 7 × 1 = 7.
Since the second cross-product is larger, the second fraction is larger.
The first cross-product is the product of the first numerator and the second denominator: 5 × 13 = 65.
The second cross-product is the product of the second numerator and the first denominator: 20 × 3 = 60.
Since the first cross-product is larger, the first fraction is larger.
Converting and Reducing Fractions
Lowest Terms
Improper Fractions
Mixed Numbers
are mixed numbers also written as 2 3/4 and 6 1/2. In these web pages, we denote mixed numbers in the form a b/c.
Converting Mixed Numbers to Improper Fractions
Converting Improper Fractions to Mixed Numbers
Writing a Fraction as a Decimal
Rounding a Fraction to the Nearest Hundredth
Adding and Subtracting Fractions
1) First, find the least common denominator.
2) Then write equivalent fractions using this denominator.
3) Add or subtract the fractions. Reduce if necessary.
Adding and Subtracting Mixed Numbers
Multiplying Fractions and Whole Numbers
Multiplying Fractions and Fractions
Multiplying Mixed Numbers
Reciprocal
Dividing Fractions
Dividing Mixed Numbers
To divide mixed numbers, you should always convert to improper fractions, then multiply the first number by the reciprocal of the second. Examples: 1 1/2 ÷ 3 1/8 = 3/2 ÷ 25/8 = 3/2 × 8/25 = (3 × 8)/(2 × 25) = 24/50 1 ÷ 3 3/5 = 1/1 ÷ 18/5 = 1/1 × 5/18 = (1 × 5)/(1 × 18) = 5/18 3 1/8 ÷ 2 = 25/8 ÷ 2/1 = 25/8 × 1/2 = (25 × 1)/(8 × 2) = 25/16 or 1 9/16. A complex fraction is a fraction whose numerator or denominator is also a fraction or mixed number. Example of complex fractions:
Simplifying Complex Fractions
otherwise written as (1/4)/(2/3), (3/7)/100, 11/(2/3), and (23 1/5)/(2/3). To simplify complex fractions, change the complex fraction into a division problem: divide the numerator by the denominator. The first of these examples becomes (1/4)/(2/3) = 1/4 ÷ 2/3 = 1/4 × 3/2 = 3/8. The second of these becomes (3/7)/100 = 3/7 ÷ 100 = 3/7 × 1/100 = 3/700. The third of these becomes 11/(2/3) = 11 ÷ 2/3 = 11 × 3/2 = 33/2 = 16 1/2. The fourth of these becomes (23 1/5)/(2/3) = 23 1/5 ÷ 2/3 = 116/5 ÷ 2/3 = 116/5 × 3/2 = 174/5 = 34 4/5.
Repeating Decimals
Every fraction can be written as a decimal. For example, 1/3 is 1 divided by 3. If you use a calculator to find 1 ÷ 3, the calculator returns 0.333333... This is called a repeating decimal. To represent the idea that the 3's repeat forever, one uses a horizontal bar (overstrike) as shown below:
Example: What is the repeating decimal for 1/7 ? Dividing 7 into 1, we get 0.142857142..., and we see the pattern begin to repeat with the second 1, so .
- Details
- Parent Category: Math League Website
Line graphsUsing Data and Statistics
Pie charts
Bar graphs
Mean
Median
Mode
Line Graphs
A line graph is a way to summarize how two pieces of information are related and how they vary depending on one another. The numbers along a side of the line graph are called the scale.
Example 1:

The graph above shows how John's weight varied from the beginning of 1991 to the beginning of 1995. The weight scale runs vertically, while the time scale is on the horizontal axis. Following the gridlines up from the beginning of the years, we see that John's weight was 68 kg in 1991, 70 kg in 1992, 74 kg in 1993, 74 kg in 1994, and 73 kg in 1995. Examining the graph also tells us that John's weight increased during 1991 and 1995, stayed the same during 1991, and fell during 1994. Example 2:

This line graph shows the average value of a pickup truck versus the mileage on the truck. When the truck is new, it costs $14000. The more the truck is driven, the more its value falls according to the curve above. Its value falls $2000 the first 20000 miles it is driven. When the mileage is 80000, the truck's value is about $4000.
Pie Charts
A pie chart is a circle graph divided into pieces, each displaying the size of some related piece of information. Pie charts are used to display the sizes of parts that make up some whole. Example 1: The pie chart below shows the ingredients used to make a sausage and mushroom pizza. The fraction of each ingredient by weight is shown in the pie chart below. We see that half of the pizza's weight comes from the crust. The mushrooms make up the smallest amount of the pizza by weight, since the slice corresponding to the mushrooms is smallest. Note that the sum of the decimal sizes of each slice is equal to 1 (the "whole" pizza"). Example 2: The pie chart below shows the ingredients used to make a sausage and mushroom pizza weighing 1.6 kg. This is the same chart as above, except that the labels no longer tell the fraction of the pizza made up by that ingredient, but the actual weight in kg of the ingredient used. The sum of the numbers shown now equals 1.6 kg, the weight of the pizza. The size of each slice is still the same, and shows us the fraction of the pizza made up from that ingredient. To get the fraction of the pizza made up by any ingredient, divide the weight of the ingredient by the weight of the pizza. What fraction of the pizza does the sausage make up? We divide 0.12 kg by 1.6 kg, to get 0.075. This is the same value as in the pie chart in the previous example.
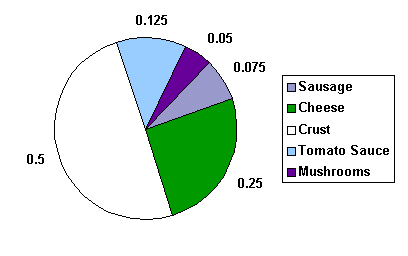
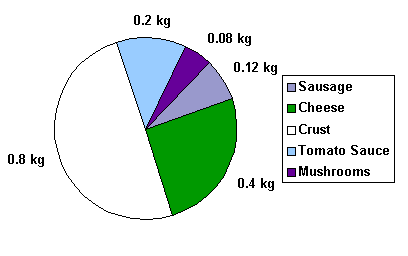
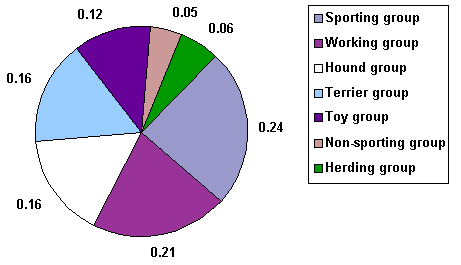
Example 3: The pie chart below shows the ingredients used to make a sausage and mushroom pizza. The fraction of each ingredient by weight shown in the pie chart below is now given as a percent. Again, we see that half of the pizza's weight, 50%, comes from the crust. Note that the sum of the percent sizes of each slice is equal to 100%. Graphically, the same information is given, but the data labels are different. Always be aware of how any chart or graph is labeled. Example 4: The pie chart below shows the fractions of dogs in a dog competition in seven different groups of dog breeds. We can see from the chart that 4 times as many dogs competed in the sporting group as in the herding group. We can also see that the two most popular groups of dogs accounted for almost half of the dogs in the competition. Suppose 1000 dogs entered the competition in all. We could figure the number of dogs in any group by multiplying the fraction of dogs in any group by 1000. In the toy group, for example, there were 0.12 × 1000 = 120 dogs in the competition.
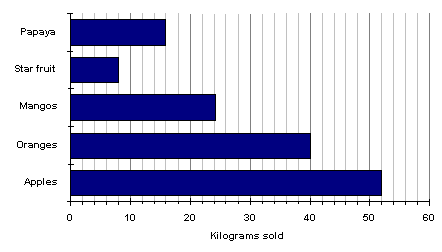
Bar graphs consist of an axis and a series of labeled horizontal or vertical bars that show different values for each bar. The numbers along a side of the bar graph are called the scale. Example 1: The bar chart below shows the weight in kilograms of some fruit sold one day by a local market. We can see that 52 kg of apples were sold, 40 kg of oranges were sold, and 8 kg of star fruit were sold.
Bar Graphs
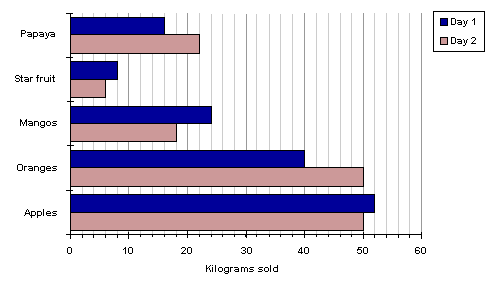
Example 2: A double bar graph is similar to a regular bar graph, but gives 2 pieces of information for each item on the vertical axis, rather than just 1. The bar chart below shows the weight in kilograms of some fruit sold on two different days by a local market. This lets us compare the sales of each fruit over a 2 day period, not just the sales of one fruit compared to another. We can see that the sales of star fruit and apples stayed most nearly the same. The sales of oranges increased from day 1 to day 2 by 10 kilograms. The same amount of apples and oranges was sold on the second day.
The mean of a list of numbers is also called the average. It is found by adding all the numbers in the list and dividing by the number of numbers in the list. Example: Find the mean of 3, 6, 11, and 8. We add all the numbers, and divide by the number of numbers in the list, which is 4. (3 + 6 + 11 + 8) ÷ 4 = 7 So the mean of these four numbers is 7. Example: Find the mean of 11, 11, 4, 10, 11, 7, and 8 to the nearest hundredth. (11 + 11 + 4 + 10 + 11 + 7 + 8) ÷ 7 = 8.857… which to the nearest hundredth rounds to 8.86. The median of a list of numbers is found by ordering them from least to greatest. If the list has an odd number of numbers, the middle number in this ordering is the median. If there is an even number of numbers, the median is the sum of the two middle numbers, divided by 2. Note that there are always as many numbers greater than or equal to the median in the list as there are less than or equal to the median in the list. Example: The students in Bjorn's class have the following ages: 4, 29, 4, 3, 4, 11, 16, 14, 17, 3. Find the median of their ages. Placed in order, the ages are 3, 3, 4, 4, 4, 11, 14, 16, 17, 29. The number of ages is 10, so the middle numbers are 4 and 11, which are the 5th and 6th entries on the ordered list. The median is the average of these two numbers: (4 + 11)/2 = 15/2 = 7.5 Example: The tallest 7 trees in a park have heights in meters of 41, 60, 47, 42, 44, 42, and 47. Find the median of their heights. Placed in order, the heights are 41, 42, 42, 44, 47, 47, 60. The number of heights is 7, so the middle number is the 4th number. We see that the median is 44.
Mean
Median
The mode in a list of numbers is the number that occurs most often, if there is one. Example: The students in Bjorn's class have the following ages: 5, 9, 1, 3, 4, 6, 6, 6, 7, 3. Find the mode of their ages. The most common number to appear on the list is 6, which appears three times. No other number appears that many times. The mode of their ages is 6.
Mode
- Details
- Parent Category: Math League Website
Decimals, Whole Numbers, and Exponents
Decimal numbers
Whole number portion
Expanded form of a decimal number
Adding decimals
Subtracting decimals
Comparing decimal numbers
Rounding decimal numbers
Estimating sums and differences
Multiplying decimal numbers
Dividing whole numbers, with remainders
Dividing whole numbers, with decimal portions
Dividing decimals by whole numbers
Dividing decimals by decimals
Exponents (powers of 2, 3, 4, ...)
Factorial notation
Square roots
Decimal Numbers
Decimal numbers such as 3.762 are used in situations which call for more precision than whole numbers provide.
As with whole numbers, a digit in a decimal number has a value which depends on the place of the digit. The places to the left of the decimal point are ones, tens, hundreds, and so on, just as with whole numbers. This table shows the decimal place value for various positions:
Note that adding extra zeros to the right of the last decimal digit does not change the value of the decimal number.
Place (underlined)
Name of Position
1.234567
Ones (units) position
1.234567
Tenths
1.234567
Hundredths
1.234567
Thousandths
1.234567
Ten thousandths
1.234567
Hundred Thousandths
1.234567
Millionths
Example:
In the number 3.762, the 3 is in the ones place, the 7 is in the tenths place, the 6 is in the hundredths place, and the 2 is in the thousandths place.
Example:
The number 14.504 is equal to 14.50400, since adding extra zeros to the right of a decimal number does not change its value.
Whole Number Portion
The whole number portion of a decimal number are those digits to the left of the decimal place.
Example:
In the number 23.65, the whole number portion is 23.
In the number 0.024, the whole number portion is 0.
Expanded Form of a Decimal Number
The expanded form of a decimal number is the number written as the sum of its whole number and decimal place values.
Example:
3 + 0.7 + 0.06 + 0.002 is the expanded form of the number 3.762.
100 + 3 + 0.06 is the expanded form of the number 103.06.
Adding Decimals
To add decimals, line up the decimal points and then follow the rules for adding or subtracting whole numbers, placing the decimal point in the same column as above.
When one number has more decimal places than another, use 0's to give them the same number of decimal places.
Example:
76.69 + 51.37
1) Line up the decimal points:
76.69
+51.37
2) Then add.
76.69
+51.37
128.06
Example:
12.924 + 3.6
1) Line up the decimal points:
12.924
+ 3.600
2) Then add.
12.924
+ 3.600
16.524
Subtracting Decimals
To subtract decimals, line up the decimal points and then follow the rules for adding or subtracting whole numbers, placing the decimal point in the same column as above.
When one number has more decimal places than another, use 0's to give them the same number of decimal places.
Example:
18.2 - 6.008
1) Line up the decimal points.
18.2
- 6.008
2) Add extra 0's, using the fact that 18.2 = 18.200
18.200
- 6.008
3) Subtract.
18.200
- 6.008
12.192
Comparing Decimal Numbers
Symbols are used to show how the size of one number compares to another. These symbols are < (less than), > (greater than), and = (equals). To compare the size of decimal numbers, we compare the whole number portions first. The larger decimal number is the one with the lager whole number portion. If the whole number parts are both equal, we compare the decimal portions of the numbers. The leftmost decimal digit is the most significant digit. Compare the pairs of digits in each decimal place, starting with the most significant digit until you find a pair that is different. The number with the larger digit is the larger number. Note that the number with the most digits is not necessarily the largest.
Example:
Compare 1 and 0.002. We begin by comparing the whole number parts: in this case 1>0, 0 being the whole number part of 0.002, and so 1>0.002.
Example:
Compare 0.402 and 0.412. The numbers 0.402 and 0.412 have the same number of digits, and their whole number parts are both 0. We compare the next most significant digit of each number, the digit in the tenths place, 4 in each case. Since they are equal, we go on to the hundredths place, and in this case, 0<1, so 0.402<0.412.
Example:
Compare 120.65 and 34.999. Comparing the whole number parts, 120>34, so 120.65>34.999.
Example:
Compare 12.345 and 12.097. Since the whole number parts are both equal, we compare the decimal portions starting with the tenths digit. Since 3>0, we have 12.345>12.097.
Note:
Remember that adding extra zeros to the right of a decimal does not change its value:
2.4 = 2.40 = 2.400 = 2.4000.
Rounding Decimal Numbers
To round a number to any decimal place value, we want to find the number with zeros in all of the lower places that is closest in value to the original number. As with whole numbers, we look at the digit to the right of the place we wish to round to. Note: When the digit 5, 6, 7, 8, or 9 appears in the ones place, round up; when the digit 0, 1, 2, 3, or 4 appears in the ones place, round down.
Examples:
Rounding 1.19 to the nearest tenth gives 1.2 (1.20).
Rounding 1.545 to the nearest hundredth gives 1.55.
Rounding 0.1024 to the nearest thousandth gives 0.102.
Rounding 1.80 to the nearest one gives 2.
Rounding 150.090 to the nearest hundred gives 200.
Rounding 4499 to the nearest thousand gives 4000.
Estimating Sums and Differences
We can use rounding to get quick estimates on sums and differences of decimal numbers. First round each number to the place value you choose, then add or subtract the rounded numbers to estimate the sum or difference.
Example:
To estimate the sum 119.36 + 0.56 to the nearest whole number, first round each number to the nearest one, giving us 119 + 1, then add to get 120.
Multiplying Decimal Numbers
Multiplying decimals is just like multiplying whole numbers. The only extra step is to decide how many digits to leave to the right of the decimal point. To do that, add the numbers of digits to the right of the decimal point in both factors.
Example:
4.032 × 4
We can multiply 4032 by 4 to get 16128. There are three decimal places in 4.032, so place the decimal three digits from the right:
4.032 × 4 = 16.128
Example:
6.74 × 9.063
We can multiply 674 by 9063 to get 6108462. Then there are 5 decimal places: two in the number 6.74 and three in the number 9.063, so place the decimal five digits from the right:
6.74 × 9.063 = 61.08462.
Dividing Whole Numbers, with Remainders
Example:
1400 ÷ 7..
Since 14 ÷ 7 = 2, and 1400 is 100 times greater than 14, the answer is 2 × 100 = 200.
Many problems are similar to the above example, where the answer is easily obtained by adding on or taking off an appropriate number of 0's. Others are more complicated.
Example:
4934 ÷ 6. Use long division.
So the answer is 822 with a remainder of 2, written 822 R2.
To double-check that the answer is correct, multiply the quotient by the divisor and add the remainder:
(822 × 6) + 2 = 4932 + 2 = 4934.
Dividing Whole Numbers, with Decimal Portions
Example:
Find 32 ÷ 6 to the nearest whole number.
32 ÷ 6 = 5 r2. 6 is the divisor; 2 is the remainder.
2 is closer to 0 than 6, so round down. The answer is 5.
Dividing Decimals by Whole Numbers
To divide a decimal by a whole number, use long division, and just remember to line up the decimal points:
Example:
13.44 ÷ 12.
When rounding an answer, divide one place further than the place you're rounding to, and round the result. Add 0's to the right of the number being divided, if necessary.
Example:
1.0 ÷ 6. Round to the nearest thousandth.
To round 0.16666 . . . to the nearest thousandth, we take 4 places to the right of the decimal point and round to 3 places. Here, we round 0.1666 to 0.167, the answer.
Dividing Decimals by Decimals
To divide by a decimal, multiply that decimal by a power of 10 great enough to obtain a whole number. Multiply the dividend by that same power of 10. Then the problem becomes one involving division by a whole number instead of division by a decimal.
Example:
0.144 ÷ 0.12
Multiplying the divisor (0.12) and the dividend (0.144) by 100, then dividing, gives the same result.
The answer is 1.2.
Be aware that some problems are less difficult and do not require this procedure.
Example:
6 ÷ 2.00
This is the same as 6 ÷ 2! The answer is 3.
Exponents (Powers of 2, 3, 4, ...)
Exponential notation is useful in situations where the same number is multiplied repeatedly. The notation is often shown as "^"
The number being multiplied is called the base, and the exponent tells how many times the base is multiplied by itself.
Example:
4 ×4 ×4 ×4 ×4 ×4 = 46
The base in this example is 4, the exponent is 6.
We refer to this as four to the sixth power, or four to the power of six, written as 4^6.
Examples:
2 ×2 ×2 = 2^3 = 8
1.1"2 = 1.1 × 1.1 = 1.21
0.5^3 = 0.5 × 0.5 × 0.5 = 0.125
10^6 = 10 × 10 × 10 × 10 × 10 × 10 = 1000000
Observe that the base may be a decimal.
Special Cases:
A number with an exponent of two is referred to as the square of a number.
The square of a whole number is known as a perfect square. The numbers 1, 4, 9, 16, and 25 are all perfect squares.
A number with an exponent of three is referred to as the cube of a number.
The cube of a whole number is known as a perfect cube. The numbers 1, 8, 27, 64, and 125 are all perfect cubes.
Note:
A number written with an exponent of 1 is the same as the given number.
23^1 = 23.
Factorial Notation n!
The product of the first n whole numbers is written as n!, and is the product
1 × 2 × 3 × 4 × … × (n - 1) × n.
Examples:
4! = 1 × 2 × 3 × 4 = 24
11! = 1 × 2 × 3 × 4 × 5 × 6 × 7 × 8 × 9 × 10 × 11 = 39916800
Tricks:
When dividing factorials, note that many of the numbers cancel out!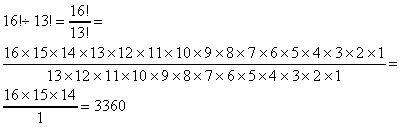
Note:
The number 0! Is defined to be 1.
Square Roots
The square root of a whole number n is the number r with the property that r × r = n.
We write this as
.
We say that the number n is the square of the number r.
Examples:
The square root of 9 is 3, since 3 × 3 = 9.
The square root of 289 is 17, since 17 × 17 = 289.
The square root of 2 is close to 1.41421. We say close to because the digits to the right of the decimal point in the square root of 2 continue forever, without any repeating pattern. Such a number is called an irrational number, meaning that it cannot be written as a fraction.
Tricks:
Since the square root of a whole number n is the number r with the property that r × r = n, we always have
That is, the square of the square root of any number is just the original number.
We also have, for any number r that the square root of the square of r is the absolute value of r.
We say the absolute value, because the notation actually means the positive square root of n.
Example:
From the example above, we see that each positive number n actually has 2 numbers r that satisfy r × r = n, one is positive, and the other is negative.
- Details
- Parent Category: Math League Website
Whole Numbers and Their Basic Properties
Using Whole Numbers
Whole numbers
Place value
Expanded form
Ordering
Rounding whole numbers
Divisibility tests
Operations and Their Properties
Commutative property of addition and multiplication
Associative property
Distributive property
The zero property of addition
The zero property of multiplication
Multiplicative identity
Order of operations
The whole numbers are the counting numbers and 0. The whole numbers are 0, 1, 2, 3, 4, 5, ...
The position, or place, of a digit in a number written in standard form determines the actual value the digit represents. This table shows the place value for various positions:
| Place (underlined) |
Name of Position |
|
1 000 |
Ones (units) position |
|
1 000 |
Tens |
|
1 000 |
Hundreds |
|
1 000 |
Thousands |
|
1 000 000 |
Ten thousands |
|
1 000 000 |
Hundred Thousands |
|
1 000 000 |
Millions |
|
1 000 000 000 |
Ten Millions |
|
1 000 000 000 |
Hundred millions |
|
1 000 000 000 |
Billions |
Example:
The number 721040 has a 7 in the hundred thousands place, a 2 in the ten thousands place, a one in the thousands place, a 4 in the tens place, and a 0 in both the hundreds and ones place.
The expanded form of a number is the sum of its various place values.
Example:
9836 = 9000 + 800 + 30 + 6.
Symbols are used to show how the size of one number compares to another. These symbols are < (less than), > (greater than), and = (equals.) For example, since 2 is smaller than 4 and 4 is larger than 2, we can write: 2 < 4, which says the same as 4 > 2 and of course, 4 = 4.
To compare two whole numbers, first put them in standard form. The one with more digits is greater than the other. If they have the same number of digits, compare the most significant digits (the leftmost digit of each number). The one having the larger significant digit is greater than the other. If the most significant digits are the same, compare the next pair of digits from the left. Repeat this until the pair of digits is different. The number with the larger digit is greater than the other.
Example: 402 has more digits than 42, so 402 > 42.
Example: 402 and 412 have the same number of digits. We compare the leftmost digit of each number: 4 in each case. Moving to the right, we compare the next two numbers: 0 and 1. Since 0 < 1, 402 < 412.
To round to the nearest ten means to find the closest number having all zeros to the right of the tens place. Note: when the digit 5, 6, 7, 8, or 9 appears in the ones place, round up; when the digit 0, 1, 2, 3, or 4 appears in the ones place, round down.
Examples:
Rounding 119 to the nearest ten gives 120.
Rounding 155 to the nearest ten gives 160.
Rounding 102 to the nearest ten gives 100.
Similarly, to round a number to any place value, we find the number with zeros in all of the places to the right of the place value being rounded to that is closest in value to the original number.
Examples:
Rounding 180 to the nearest hundred gives 200.
Rounding 150090 to the nearest hundred thousand gives 200000.
Rounding 1234 to the nearest thousand gives 1000.
Rounding is useful in making estimates of sums, differences, etc.
Example:
To estimate the sum 119360 + 500 to the nearest thousand, first round each number in the sum, resulting in a new sum of 119000 + 1000.. Then add to get the estimate of 120000.
There are many quick ways of telling whether or not a whole number is divisible by certain basic whole numbers. These can be useful tricks, especially for large numbers.
| Divisibility by 2 |
Commutative Property of Addition and Multiplication
Addition and Multiplication are commutative: switching the order of two numbers being added or multiplied does not change the result.
Examples:
100 + 8 = 8 + 100
100 × 8 = 8 × 100
Addition and multiplication are associative: the order that numbers are grouped in addition and multiplication does not affect the result.
Examples:
(2 + 10) + 6 = 2 + (10 + 6) = 18
2 × (10 × 6) = (2 × 10) × 6 =120
The distributive property of multiplication over addition: multiplication may be distributed over addition.
Examples:
10 × (50 + 3) = (10 × 50) + (10 × 3)
3 × (12+99) = (3 × 12) + (3 × 99)
Adding 0 to a number leaves it unchanged. We call 0 the additive identity.
Example:
88 + 0 = 88
The Zero Property of Multiplication
Multiplying any number by 0 gives 0.
Example:
88 × 0 = 0
0 × 1003 = 0
We call 1 the multiplicative identity. Multiplying any number by 1 leaves the number unchanged.
Example:
88 × 1 = 88
The order of operations for complicated calculations is as follows:
1) Perform operations within parentheses.
2) Multiply and divide, whichever comes first, from left to right.
3) Add and subtract, whichever comes first, from left to right.
Example:
1 + 20 × (6 + 2) ÷ 2 =
1 + 20 × 8 ÷ 2 =
1 + 160 ÷ 2 =
1 + 80 =
81.
A whole number is divisible by 2 if the digit in its units position is even, (either 0, 2, 4, 6, or 8).
Examples:
The number 84 is divisible by 2 since the digit in the units position is 4, which is even.
The number 333336 is divisible by 2 since the digit in the units position is 6, which is even.
The number 1297000 is divisible by 2 since the digit in the units position is 0, which is even.
A whole number is divisible by 3 if the sum of all its digits is divisible by 3.
Examples:
The number 177 is divisible by three, since the sum of its digits is 15, which is divisible by 3.
The number 8882151 is divisible by three, since the sum of its digits is 33, which is divisible by 3.
The number 162345 is divisible by three, since the sum of its digits is 21, which is divisible by 3.
If a number is not divisible by 3, the remainder when it is divided by 3 is the same as the remainder when the sum of its digits is divided by 3.
Examples:
The number 3248 is not divisible by 3, since the sum of its digits is 17, which is not divisible by 3. When 3248 is divided by 3, the remainder is 2, since when 17, the sum of its digits, is divided by three, the remainder is 2.
The number 172345 is not divisible by 3, since the sum of its digits is 22, which is not divisible by 3. When 172345 is divided by 3, the remainder is 1, since when 22, the sum of its digits, is divided by three, the remainder is 1.
A whole number is divisible by 4 if the number formed by the last two digits is divisible by 4.
Examples:
The number 3124 is divisible by 4 since the number formed by its last two digits, 24, is divisible by 4.
The number 1333336 is divisible by 4 since the number formed by its last two digits, 36, is divisible by 4.
The number 1297000 is divisible by 4 since the number formed by its last two digits, 0, is divisible by 4.
If a number is not divisible by 4, the remainder when the number is divided by 4 is the same as the remainder when the last two digits are divided by 4.
Example:
The number 172345 is not divisible by 4, since the number formed by its last two digits, 45, is not divisible by 4. When 172345 is divided by 4, the remainder is 1, since when 45 is divided by 4, the remainder is 1.
A whole number is divisible by 5 if the digit in its units position is 0 or 5.
Examples:
The number 95 is divisible by 5 since the last digit is 5.
The number 343370 is divisible by 5 since the last digit is 0.
The number 129700195 is divisible by 5 since the last digit is 5.
If a number is not divisible by 5, the remainder when it is divided by 5 is the same as the remainder when the last digit is divided by 5.
Example:
The number 145632 is not divisible by 5, since the last digit is 2. When 145632 is divided by 5, the remainder is 2, since 2 divided by 5 is 0 with a remainder of 2.
The number 7332899 is not divisible by 5, since the last digit is 9. When 7332899 is divided by 5, the remainder is 4, since 9 divided by 5 is 1 with a remainder of 4.
A number is divisible by 6 if it is divisible by 2 and divisible by 3. We can use each of the divisibility tests to check if a number is divisible by 6: its units digit is even and the sum of its digits is divisible by 3.
Examples:
The number 714558 is divisible by 6, since its units digit is even, and the sum of its digits is 30, which is divisible by 3.
The number 297663 is not divisible by 6, since its units digit is not even.
The number 367942 is not divisible by 6, since it is not divisible by 3. The sum of its digits is 31, which is not divisible by 3, so the number 367942 is not divisible by 3.
A whole number is divisible by 8 if the number formed by the last three digits is divisible by 8.
Examples:
The number 88863024 is divisible by 8 since the number formed by its last three digits, 24, is divisible by 8.
The number 17723000 is divisible by 8 since the number formed by its last three digits, 0, is divisible by 8.
The number 339122483984 is divisible by 8 since the number formed by its last three digits, 984, is divisible by 8.
If a number is not divisible by 8, the remainder when the number is divided by 8 is the same as the remainder when the last three digits are divided by 8.
Example:
The number 172045 is not divisible by 8, since the number formed by its last three digits, 45, is not divisible by 8. When 172345 is divided by 8, the remainder is 5, since when 45 is divided by 8, the remainder is 5.
A whole number is divisible by 9 if the sum of all its digits is divisible by 9.
Examples:
The number 1737 is divisible by nine, since the sum of its digits is 18, which is divisible by 9.
The number 8882451 is divisible by nine, since the sum of its digits is 36, which is divisible by 9.
The number 762345 is divisible by nine, since the sum of its digits is 27, which is divisible by 9.
If a number is not divisible by 9, the remainder when it is divided by 9 is the same as the remainder when the sum of its digits is divided by 9.
Examples:
The number 3248 is not divisible by 9, since the sum of its digits is 17, which is not divisible by 9. When 3248 is divided by 9, the remainder is 8, since when 17, the sum of its digits, is divided by 9, the remainder is 8.
The number 172345 is not divisible by 9, since the sum of its digits is 22, which is not divisible by 9. When 172345 is divided by 9, the remainder is 4, since when 22, the sum of its digits, is divided by 9, the remainder is 4.
A whole number is divisible by 10 if the digit in its units position is 0.
Examples:
The number 1229570 is divisible by 10 since the last digit is 0.
The number 676767000 is divisible by 10 since the last digit is 0.
The number 129700190 is divisible by 10 since the last digit is 0.
If a number is not divisible by 10, the remainder when it is divided by 10 is the same as the units digit.
Examples:
The number 145632 is not divisible by 10, since the last digit is 2. When 145632 is divided by 10, the remainder is 2, since the units digit is 2.
The number 7332899 is not divisible by 10, since the last digit is 9. When 7332899 is divided by 10, the remainder is 4, since the units digit is 9.
Starting with the units digit, add every other digit and remember this number. Form a new number by adding the digits that remain. If the difference between these two numbers is divisible by 11, then the original number is divisible by 11.
Examples:
Is the number 824472 divisible by 11? Starting with the units digit, add every other number:2 + 4 + 2 = 8. Then add the remaining numbers: 7 + 4 + 8 = 19. Since the difference between these two sums is 11, which is divisible by 11, 824472 is divisible by 11.
Is the number 49137 divisible by 11? Starting with the units digit, add every other number:7 + 1 + 4 = 12. Then add the remaining numbers: 3 + 9 = 12. Since the difference between these two sums is 0, which is divisible by 11, 49137 is divisible by 11.
Is the number 16370706 divisible by 11? Starting with the units digit, add every other number:6 + 7 + 7 + 6 = 26. Then add the remaining numbers: 0 + 0 + 3 + 1=4. Since the difference between these two sums is 22, which is divisible by 11, 16370706 is divisible by 11.
A number is divisible by 12 if it is divisible by 4 and divisible by 3. We can use each of the divisibility tests to check if a number is divisible by 12: its last two digits are divisible by 4 and the sum of its digits is divisible by 3.
Examples:
The number 724560 is divisible by 12, since the number formed by its last two digits, 60, is divisible by 4, and the sum of its digits is 30, which is divisible by 3.
The number 36297414 is not divisible by 12, since the number formed by its last two digits, 14, is not divisible by 4.
The number 367744 is not divisible by 12, since it is not divisible by 3. The sum of its digits is 29, which is not divisible by 3, so the number 367942 is not divisible by 3.
A number is divisible by 15 if it is divisible by 3 and divisible by 5. We can use each of the divisibility tests to check if a number is divisible by 15: its units digit is 0 or 5, and the sum of its digits is divisible by 3.
Example:
The number 7145580 is divisible by 15, since its units digit is even, and the sum of its digits is 30, which is divisible by 3.
A whole number is divisible by 16 if the number formed by the last four digits is divisible by 16.
Examples:
The number 898630032 is divisible by 16 since the number formed by its last four digits, 32, is divisible by 16.
The number 1772300000 is divisible by 16 since the number formed by its last four digits, 0, is divisible by 16.
The number 339122481296 is divisible by 16 since the number formed by its last four digits, 1296, is divisible by 16.
If a number is not divisible by 16, the remainder when the number is divided by 16 is the same as the remainder when the last four digits are divided by 16.
Example:
The number 172411045 is not divisible by 16, since the number formed by its last four digits, 1045, is not divisible by 16. When 172411045 is divided by 16, the remainder is 5, since when 1045 is divided by 16, the remainder is 5.
A number is divisible by 18 if it is divisible by 2 and divisible by 9. We can use each of the divisibility tests to check if a number is divisible by 18: its units digit is even and the sum of its digits is divisible by 9.
Examples:
The number 7145586 is divisible by 18, since its units digit is even, and the sum of its digits is 36, which is divisible by 9.
The number 2976633 is not divisible by 18, since its units digit is not even.
The number 367942 is not divisible by 18, since it is not divisible by 9. The sum of its digits is 31, which is not divisible by 9, so the number 367942 is not divisible by 9.
A number is divisible by 20 if its units digit is 0, and its tens digit is even. In other words, the last two digits form one of the numbers 0, 20, 40, 60, or 80.
Examples:
The number 3351002760 is divisible by 20, since the number formed by its last two digits is 60.
The number 802199730000 is divisible by 20, since the number formed by its last two digits is 0.
A number is divisible by 22 if it is divisible by the numbers 2 and 11. We can use each of the divisibility tests to check if a number is divisible by 22: its units digit is even, and the difference between the sums of every other digit is divisible by 11.
Example:
Is the number 117524 divisible by 22? The units digit is even, so it is divisible by 2. The two sums of every other digit are 4 + 5 + 1 = 10 and 2 + 7 + 1 = 10, which have a difference of 0. Since 0 is divisible by 11, 117524 is divisible by 11. Thus, 117524 is divisible by 22, since it is divisible by both 2 and 11.
A number is divisible by 25 if the number formed by the last two digits is any of 0, 25, 50, or 75 (the number formed by its last two digits is divisible by 25).
Examples:
The number 73224050 is divisible by 25, since its last two digits form the number 50.
The number 1008922200 is divisible by 25, since its last two digits form the number 0.
- Details
- Parent Category: Math League Website
This is a help resource for 4th through 8th grades. We have just redesigned and reformatted these pages to enable faster loading and display times, but some pages still load slowly. Different browsers display graphics differently, so some math symbols may not appear to line up perfectly in some sentences. Our educational CD-ROMs contain the complete, searchable, indexed help facility shown below, along with the helpful problem-solving tips section. We hope you find this a helpful resource.
Whole numbers and their basic properties
Decimals, whole numbers, and exponents
Using data and statistics
Fractions
Geometry
Ratio and proportion
Percent and probability
Integers
Metric units and measurement
Introduction to algebra
Positive and negative numbers
- Details
- Parent Category: Math League Website
MATHEMATICS LEAGUE
4TH, 5TH, 6TH, 7TH, 8TH GRADE
and ALGEBRA COURSE 1 CONTEST ORGANIZATION
QUESTIONS (TIME LIMITS AND TOPICS) Each contest is a 30-minute multiple-choice test. Questions may involve any topic appropriate to the grade level of the contest. If, for any reason, a question must be dropped, no replacement will be made.
CONTEST COPIES Each school will receive 30 copies of each contest in which they are participating. Schools requiring additional copies of any contest are permitted, on the day of the contest, to make as many additional copies as are required. A separate registration form should be submitted from each participating school.
CONTEST MATERIALS PACKAGE You should receive materials for Algebra Course 1 and Grades 4 & 5 by April 1; for Grade 6 by the last Tuesday in February; for Grades 7 & 8 by the next to the last Tuesday in January. If any contest materials have not been received by these dates, the League should be phoned immediately at 1-201-568-6328. Each contest materials package includes 30 copies of the contest and a solution key for the contest. A school needing additional copies of any contest is permitted, on the day of the contest, to make as many additional copies of the contest as are required. Special arrangements for blind or other handicapped students, or for non-English-speaking students, may be made by any school.
CONTEST AWARDS
GRADES 4 AND 5 and ALGEBRA COURSE 1 In each school, the highest scoring student on each contest receives a book award. Other high scoring students in each school receive certificates of merit.
GRADES 6, 7, AND 8 In each school, a certificate of merit is awarded to the highest scoring student on each contest. For each contest, awards are given to the two schools with the highest total scores in the League and also to the two students with the highest total scores in the League. For each contest, additional regional awards are given to the highest scoring school in each region (which may be a county, province, state or other grouping as determined by the League). Counties/Provinces/States with fewer than fifteen participating schools are grouped together into regions for the purposes of awards. No school may win both a regional award and an overall League award on any one grade level in the same school year. The League reserves the right to break ties based upon performance on selected questions, or, at its option, to issue duplicate awards.
CONTEST LOCATION Each school may administer the contests on its own premises or any other suitable site.
6TH, 7TH, AND 8TH GRADE CONTEST PROCEDURES
(The 4th and 5th Grade and Algebra Course 1 Contests are non-competitive; these procedures do not apply.)
CONTEST DATE Except in unusual circumstances, the contests must be held on the scheduled date. In the event of school closings, special testing days, school trips or other administrative functions, severely inclement weather, or similar disruptions of the normal school day, permission is granted to conduct the contest on a proximate school day.
STARTING TIME Each contest may be held, on the scheduled date, at any time convenient for the school. All students officially participating in a contest within the same school should take that contest at the same time. Scores of students taking the contest at any later time should not be included on the score report filed with the League.
PROCTORING Each contest must be actively proctored at all times by a teacher. Neither the proctor nor anyone else may interpret any question to any student during the contest.
ELIGIBILITY Only students officially registered in the same accredited school of record may participate on that school's team. A student may take only a contest designed for a grade the student has not completed or a higher grade (regardless of the math course in which the student is enrolled.) Students taking the 4th or 5th grade contest may also take the 6th, 7th, or 8th grade contest. Students taking the 6th, 7th, or 8th grade contest may take only one such contest (but they may take the Algebra Course 1 Contest). On each contest, all official participants must take the contest in school at the exact same time. A student taking the contest at a later time or period or on a later day must not be included on the score report. Students absent on the contest day may take the contest but must not be listed on the score report.
MATERIALS ALLOWED Only plain paper, pencil or pen, and any calculator without a QWERTY keyboard, may be used by the participants. No graph paper, compasses, straight edges, rulers, printed mathematical tables, or other devices shall be allowed, except where special arrangements have been made for handicapped students or when dictionaries are made accessible to non-English-speaking students.
START OF THE CONTEST Each contestant should be given a copy of the contest and should complete the information requested on the cover page of the contest. Answers submitted for each question must appear in the appropriate space in the answer column. Answers written elsewhere will receive no credit. After the signal to begin is given by the proctor, the timing of the contest will begin.
TIME WARNINGS Warnings that "fifteen minutes remain," that "five minutes remain," and that "one minute remains" should be given at the appropriate times. No other warnings or announcements (relative to the contest) should be made to any contestant during the contest.
MARKING THE ANSWERS At the end of the contest, the question papers should be collected by the advisor. The advisor should then open the sealed envelope containing the solution key and should mark each paper, awarding 1 point for each correct answer. All papers should be marked exactly according to the solution key. If you wish to appeal an answer, please follow the appeals procedure, but score your students' papers according to the official answer key. The League has the option to disqualify any school that submits a mismarked paper.
SUBMITTING CONTEST RESULTS ONLINE The advisor should score the contests. For each contest, the advisor should submit the scores of the school's participants to the League's Internet Score Report Center. The school score for each contest will be the sum of the scores of the five highest scoring participants from the same school of record. The score report must be submitted to our Internet Score Report Center by Friday of the contest week.Student papers may be returned to the students, except that papers with scores above 30 must be held until June 1.
APPEALS PROCEDURE Appeals will be awarded only on the basis of an incorrect official answer or a correct alternative interpretation of a question. Detailed explanations of alternative interpretations should be made in the comments section when filing the score reports. Appeals filed with the League must include the names of all students listed on the score report for whom an appeal is being filed. If you disagree with an official answer, file an appeal. You must use only the official solution key in grading student papers.
AUTHENTICATION OF RESULTS League policy is to authenticate scores and eligibility of participants from schools winning major awards. The League reserves the right to authenticate scores and/or to reexamine students or validate student solutions before granting official status to any score. Schools must keep all papers with scores above 30 until June 1. The League has the option to disqualify any school that submits a mismarked paper. A school disqualified for cause on any contest is ineligible for awards in any League contest.
Directors:
Dan Flegler / Phone: 1-201-568-6328, Fax: 1-201-816-0125
Steve Conrad / Phone: 1-516-365-5656, Fax: 1-516-365-5657
- Details
- Parent Category: Math League Website
How to get your school involved in Math League Contests
Check the Math League Registration Center for the Math League Contests in your state to see if your school is participating, and in which contests. Schools are listed by County in the U.S., and by Province in Canada. If your school is not yet registered, and you would like to see them compete in Math League Contests, you can encourage them to participate. Here's how:
|
- Details
- Parent Category: Math League Website
The Math League was formed in 1977 by Steven R. Conrad and Daniel Flegler.
In October 1985, Steven R. Conrad and Daniel Flegler were both honored by President Ronald Reagan as recipients of Presidential awards for "Excellence in Mathematics Teaching." Mr. Conrad was the winner from New York, and Mr. Flegler was the winner from New Jersey. Mr. Flegler was the 1977 recipient of Princeton University's award for "Distinguished Secondary School Teaching." Mr. Conrad and Mr. Flegler have been preparing contests for math students across North America since 1977. They have co-authored 18 books.
Steven R. Conrad taught at Roslyn High School, Roslyn, New York, from 1980 to 1998. Prior to that, he taught at Benjamin Cardozo High School in Bayside, New York and Francis Lewis High School in Flushing, New York.
He began his undergraduate education at Washington University, St. Louis. He received a B.S. from Queens College and an M.S. from Yeshiva University. He has done additional graduate study at the University of San Francisco, Fordham University, and St. John's University, where he earned a certificate in School Administration.
More than 60 of Mr. Conrad's students have been named to the honors group of the Intel National Science Talent Search for mathematics papers they have written. Six of them have finished in the top 10 nationwide. His sons are both math professors.
He was Problem Editor for The Mathematics Student Journal (an official journal of the National Council of Teachers of Mathematics) from 1972 to 1978 and was an associate editor of The New York State Mathematics Teachers' Journal for 3 years. He has contributed problems to many journals, and has had articles published in Mathematics Magazine, The Mathematics Teacher, , American Mathematical Monthly, and Crux Mathematicorum. He is a reviewer for The Mathematics Teacher. For 4 years, Mr. Conrad was the author for the American Regions Mathematics League. He has also been the contest author for the Association of Mathematics Teachers of New Jersey, and director of contests for New York City. Currently, he is the author for contests sponsored by Bergen County, New Jersey, Suffolk County, New York, and Fairfax County, Virginia. He has also authored contests for Montgomery County, Maryland and Nassau County, New York. County, New York. Mr. Conrad served for six years as a member of the committee which developed the SAT II for the College Board.
Daniel Flegler taught at Waldwick High School, Waldwick, New Jersey, from 1965 to 1991, where he served as department chairman for 11 years. Mr. Flegler received his B.A. from Brown University and his M.A. from Columbia University. He has done additional graduate work at Fairleigh Dickinson University, the University of Iowa, and Rutgers University, from which he received his certificate in educational administration.
More than 15 of Mr. Flegler's students have been named to the honors group of the Intel National Science Talent Search for mathematics papers they have written. Three of them have finished in the top 40 nationwide.
From 1972-78, Mr. Flegler served on the Executive Committee of the Association of Mathematics Teachers of New Jersey, and was assistant editor of the New Jersey Mathematics Teacher. In addition, he has written contest problems for both New York City and Nassau County, New York. He has also directed the contests for Bergen County, New Jersey.




