Angles and Angle Terms

Angles and angle terms

What is an angle?
Degrees: measuring angles
Acute angles
Obtuse angles
Right angles
Complementary angles
Supplementary angles
Vertical angles
Alternate interior angles
Alternate exterior angles
Corresponding angles
Angle bisector
Perpendicular lines

What is an Angle?

Two rays that share the same endpoint form an angle. The point where the rays intersect is called the vertex of the angle. The two rays are called the sides of the angle.

Example: Here are some examples of angles.

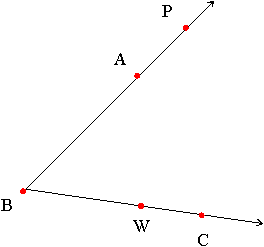
We can specify an angle by using a point on each ray and the vertex. The angle below may be specified as angle ABC or as angle CBA; you may also see this written as 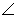ABC or as 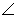CBA. Note how the vertex point is always given in the middle.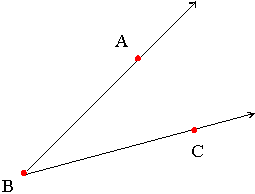

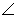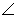Example: Many different names exist for the same angle. For the angle below, PBC, PBW, 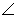CBP, and 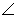WBA are all names for the same angle.

Degrees: Measuring Angles

We measure the size of an angle using degrees.

Example: Here are some examples of angles and their degree measurements.

Acute Angles

An acute angle is an angle measuring between 0 and 90 degrees.

Example:

The following angles are all acute angles.

Obtuse Angles

An obtuse angle is an angle measuring between 90 and 180 degrees.

Example:

The following angles are all obtuse.

Right Angles

A right angle is an angle measuring 90 degrees. Two lines or line segments that meet at a right angle are said to be perpendicular. Note that any two right angles are supplementary angles (a right angle is its own angle supplement).

Example:

The following angles are both right angles.

Complementary Angles

Two angles are called complementary angles if the sum of their degree measurements equals 90 degrees. One of the complementary angles is said to be the complement of the other.

Example:

These two angles are complementary.

Note that these two angles can be "pasted" together to form a right angle!

Supplementary Angles

Two angles are called supplementary angles if the sum of their degree measurements equals 180 degrees. One of the supplementary angles is said to be the supplement of the other.

Example:

These two angles are supplementary.

Note that these two angles can be "pasted" together to form a straight line!

Vertical Angles

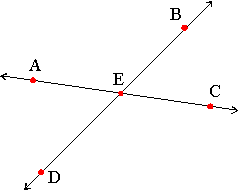
For any two lines that meet, such as in the diagram below, angle AEB and angle DEC are called vertical angles. Vertical angles have the same degree measurement. Angle BEC and angle AED are also vertical angles.

Alternate Interior Angles

For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle D are called alternate interior angles. Alternate interior angles have the same degree measurement. Angle B and angle C are also alternate interior angles.

Alternate Exterior Angles

For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle D are called alternate exterior angles. Alternate exterior angles have the same degree measurement. Angle B and angle C are also alternate exterior angles.

Corresponding Angles

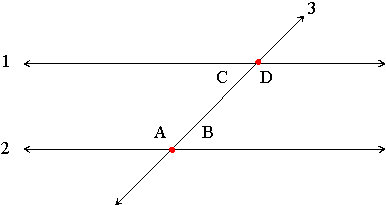
For any pair of parallel lines 1 and 2, that are both intersected by a third line, such as line 3 in the diagram below, angle A and angle C are called corresponding angles. Corresponding angles have the same degree measurement. Angle B and angle D are also corresponding angles.

Angle Bisector

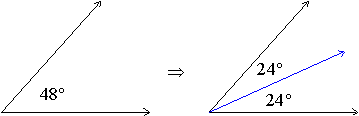
An angle bisector is a ray that divides an angle into two equal angles.

Example:

The blue ray on the right is the angle bisector of the angle on the left.

The red ray on the right is the angle bisector of the angle on the left.

Perpendicular Lines

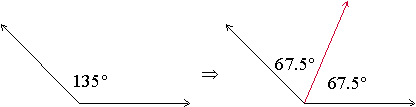
Two lines that meet at a right angle are perpendicular.