Coordinates and Similar Figures

Coordinates and similar figures

What is a coordinate?
Similar figures
Congruent figures
Rotation
Reflection
Folding
Symmetric figure

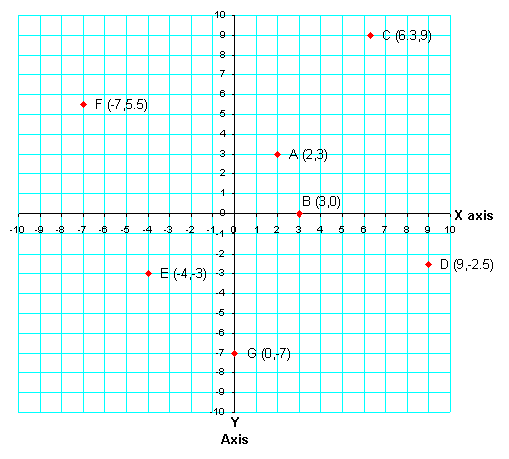
What is a Coordinate?

Coordinates are pairs of numbers that are used to determine points in a plane, relative to a special point called the origin. The origin has coordinates (0,0). We can think of the origin as the center of the plane or the starting point for finding all other points. Any other point in the plane has a pair of coordinates (x,y). The x value or x-coordinate tells how far left or right the point is from the point (0,0), just like on the number line (negative is left of the origin, positive is right of the origin). The y value or y-coordinate tells how far up or down the point is from the point (0,0), (negative is down from the origin, positive is up from the origin). Using coordinates, we may give the location of any point we like by simply using a pair of numbers.

Example:

The origin below is where the x-axis and the y-axis meet. Point A has coordinates (2,3), since it is 2 units to the right and 3 units up from the origin. Point B has coordinates (3,0), since it is 3 units to the right, and lies on the x-axis. Point C has coordinates (6.3,9), since it is 6.3 units to the right, and 9 units up from the origin. Point D has coordinates (9,-2.5); it is 9 units to the right, and 2.5 units down from the origin. Point E has coordinates (-4,-3); it is 4 units to the left, and 3 units down from the origin. Point F has coordinates (-7,5.5); it is 7 units to the left, and 6 units up from the origin. Point G has coordinates (0,-7) since it lies on the y-axis 7 units below the origin.

Similar Figures

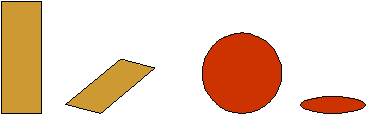
Figures that have the same shape are called similar figures. They may be different sizes or turned somewhat.

Example:

The following pairs of figures are similar.

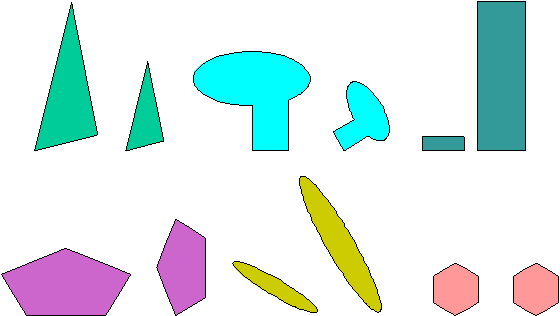
These pairs of figures are not similar.

Congruent Figures

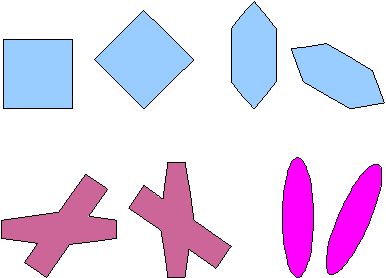
Two figures are congruent if they have the same shape and size.

Example:

The following pairs of figures below are congruent. Note that if two figures are congruent, they must be similar.

The pairs below are similar but not congruent.

The pairs below are not similar or congruent.

Rotation

When a figure is turned, we call it a rotation of the figure. We can measure this rotation in terms of degrees; a 360 degree turn rotates a figure around once back to its original position.

Example:

For the following pairs of figures, the figure on the right is a rotation of the figure on the left.

Reflection

If we flip (or mirror) along some line, we say the figure is a reflection along that line.

Examples:

Reflections along a vertical line:

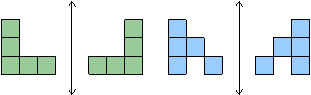
Reflections along a horizontal line:

Reflections along a diagonal line:

Folding

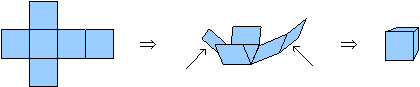
When we talk about folding a plane figure, we mean folding it as if it were a piece of paper in that shape. We might fold this into a solid figure such as a box, or fold the figure flat along itself.

Example:

Folding the figure on the left into a box:

Folding the figure on the left flat along the dotted line:

Symmetric Figure

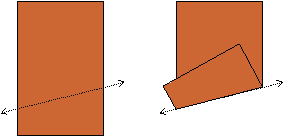
A figure that can be folded flat along a line so that the two halves match perfectly is a symmetric figure; such a line is called a line of symmetry.

Examples:

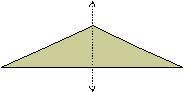
The triangle below is a symmetric figure. The dotted line is the line of symmetry.

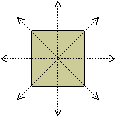
The square below is a symmetric figure. It has four different lines of symmetry shown below.

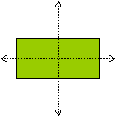
The rectangle below is a symmetric figure. It has two different lines of symmetry shown below.

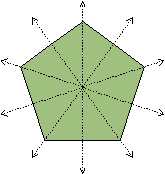
The regular pentagon below is a symmetric figure. It has five different lines of symmetry shown below.

The circle below is a symmetric figure. Any line that passes through its center is a line of symmetry!

The figures shown below are not symmetric.