Positive and Negative Numbers

Positive and negative numbers

About positive and negative numbers
The number line
Absolute value of positive and negative numbers
Adding positive and negative numbers
Subtracting positive and negative numbers
Multiplying positive and negative numbers
Dividing positive and negative numbers
Coordinates
Comparing positive and negative numbers
Reciprocals of negative numbers

About Positive and Negative Numbers

Positive numbers are any numbers greater than zero, for example: 1, 2.9, 3.14159, 40000, and 0.0005. For each positive number, there is a negative number that is its opposite. We write the opposite of a positive number with a negative or minus sign in front of the number, and call these numbers negative numbers. The opposites of the numbers in the list above would be: -1, -2.9, -3.14159, -40000, and -0.0005. Negative numbers are less than zero (see the number line for a more complete explanation of this). Similarly, the opposite of any negative number is a positive number. For example, the opposite of -12.3 is 12.3.
We do not consider zero to be a positive or negative number. 
The sum of any number and its opposite is 0.
The sign of a number refers to whether the number is positive or negative, for example, the sign of -3.2 is negative, and the sign of 442 is positive.
We may also write positive and negative numbers as fractions or mixed numbers.

The following fractions are all equal:

(-1)/3, 1/(-3), -(1/3) and - 1/3.

The following mixed numbers are all equal:

-1 1/6, -(1 1/6), (-7)/6, 7/(-6), and - 7/6.

The Number Line

The number line is a line labeled with positive and negative numbers in increasing order from left to right, that extends in both directions. The number line shown below is just a small piece of the number line from -4 to 4.

For any two different places on the number line, the number on the right is greater than the number on the left.

Examples:

4 > -2, 1 > -0.5, -2 > -4, and 0 > -15

Absolute Value of Positive and Negative Numbers

The number of units a number is from zero on the number line. The absolute value of a number is always a positive number (or zero). We specify the absolute value of a number n by writing n in between two vertical bars: |n|.

Examples:

|6| = 6
|-0.004| = 0.004
|0| = 0
|3.44| = 3.44
|-3.44| = 3.44
|-10000.9| = 10000.9

Adding Positive and Negative Numbers

1) When adding numbers of the same sign, we add their absolute values, and give the result the same sign.

Examples:

2 + 5.7 = 7.7
(-7.3) + (-2.1) = -(7.3 + 2.1) = -9.4 
(-100) + (-0.05) = -(100 + 0.05) = -100.05

2) When adding numbers of the opposite signs, we take their absolute values, subtract the smaller from the larger, and give the result the sign of the number with the larger absolute value.

Example:

7 + (-3.4) = ?
The absolute values of 7 and -3.4 are 7 and 3.4. Subtracting the smaller from the larger gives 7 - 3.4 = 3.6, and since the larger absolute value was 7, we give the result the same sign as 7, so 7 + (-3.4) = 3.6.

Example:

8.5 + (-17) = ? 
The absolute values of 8.5 and -17 are 8.5 and 17. Subtracting the smaller from the larger gives 17 - 8.5 = 8.5, and since the larger absolute value was 17, we give the result the same sign as -17, so 8.5 + (-17) = -8.5.

Example:

-2.2 + 1.1 = ?
The absolute values of -2.2 and 1.1 are 2.2 and 1.1. Subtracting the smaller from the larger gives 2.2 - 1.1 = 1.1, and since the larger absolute value was 2.2, we give the result the same sign as -2.2, so -2.2 + 1.1 = -1.1.

Example:

6.93 + (-6.93) = ?
The absolute values of 6.93 and -6.93 are 6.93 and 6.93. Subtracting the smaller from the larger gives 6.93 - 6.93 = 0. The sign in this case does not matter, since 0 and -0 are the same. Note that 6.93 and -6.93 are opposite numbers. All opposite numbers have this property that their sum is equal to zero. Two numbers that add up to zero are also called additive inverses.

Subtracting Positive and Negative Numbers

Subtracting a number is the same as adding its opposite.

Examples:

In the following examples, we convert the subtracted number to its opposite, and add the two numbers.
7 - 4.4 = 7 + (-4.4) = 2.6 
22.7 - (-5) = 22.7 + (5) = 27.7 
-8.9 - 1.7 = -8.9 + (-1.7) = -10.6 
-6 - (-100.6) = -6 + (100.6) = 94.6

Note that the result of subtracting two numbers can be positive or negative, or 0.

Multiplying Positive and Negative Numbers

To multiply a pair of numbers if both numbers have the same sign, their product is the product of their absolute values (their product is positive). If the numbers have opposite signs, their product is the opposite of the product of their absolute values (their product is negative). If one or both of the numbers is 0, the product is 0.

Examples:

In the product below, both numbers are positive, so we just take their product.
0.5 × 3 = 1.5

In the product below, both numbers are negative, so we take the product of their absolute values.
(-1.1) × (-5) = |-1.1| × |-5| = 1.1 × 5 = 5.5

In the product of (-3) × 0.7, the first number is negative and the second is positive, so we take the product of their absolute values, which is |-3| × |0.7| = 3 × 0.7 = 2.1, and give this result a negative sign: -2.1, so (-3) × 0.7 = -2.1

In the product of 21 × (-3.1), the first number is positive and the second is negative, so we take the product of their absolute values, which is |21| × |-3.1| = 21 × 3.1 = 65.1, and give this result a negative sign: -65.1, so 21 × (-3.1) = -65.1.

To multiply any number of numbers:

1. Count the number of negative numbers in the product. 
2. Take the product of their absolute values.
3. If the number of negative numbers counted in step 1 is even, the product is just the product from step 2, if the number of negative numbers is odd, the product is the opposite of the product in step 2 (give the product in step 2 a negative sign). If any of the numbers in the product is 0, the product is 0.

Example:

2 × (-1.1) × 5 (-1.2) × (-9) = ? 
Counting the number of negative numbers in the product, we see that there are 3 negative numbers: -1.1, -1.2, and -9. Next, we take the product of the absolute values of each number: 2 × |-1.1| × 5 × |-1.2| × |-9| = 2 × 1.1 × 5 × 1.2 × 9 = 118.8 
Since there were an odd number of numbers, the product is the opposite of 118.8, which is -118.8, so 2 × (-1.1) × 5 (-1.2) × (-9) = -118.8.

Dividing Positive and Negative Numbers

To divide a pair of numbers if both numbers have the same sign, divide the absolute value of the first number by the absolute value of the second number.
To divide a pair of numbers if both numbers have different signs, divide the absolute value of the first number by the absolute value of the second number, and give this result a negative sign.

Examples:

In the division below, both numbers are positive, so we just divide as usual.
7 ÷ 2 = 3.5

In the division below, both numbers are negative, so we divide the absolute value of the first by the absolute value of the second. 
(-2.4) ÷ (-3) = |-2.4| ÷ |-3| = 2.4 ÷ 3 = 0.8

In the division (-1) ÷ 2.5, both number have different signs, so we divide the absolute value of the first number by the absolute value of the second, which is |-1| ÷ |2.5| = 1 ÷ 2.5 = 0.4, and give this result a negative sign: -0.4, so (-1) ÷ 2.5 = -0.4.

In the division 9.8 ÷ (-0.7), both number have different signs, so we divide the absolute value of the first number by the absolute value of the second, which is |9.8| ÷ |-0.7| = 9.8 ÷ 0.7 = 14, and give this result a negative sign: -14, so 9.8 ÷ (-0.7) = -14.

Coordinates

Number coordinates are pairs of numbers that are used to determine points in a grid, relative to a special point called the origin. The origin has coordinates (0,0). We can think of the origin as the center of the grid or the starting point for finding all other points. Any other point in the grid has a pair of coordinates (x,y). The x value or x-coordinate tells how many steps left or right the point is from the point (0,0), just like on the number line (negative is left of the origin, positive is right of the origin). The y value or y-coordinate tells how many steps up or down the point is from the point (0,0), (negative is down from the origin, positive is up from the origin). Using coordinates, we may give the location of any point in the grid we like by simply using a pair of numbers.

Example:

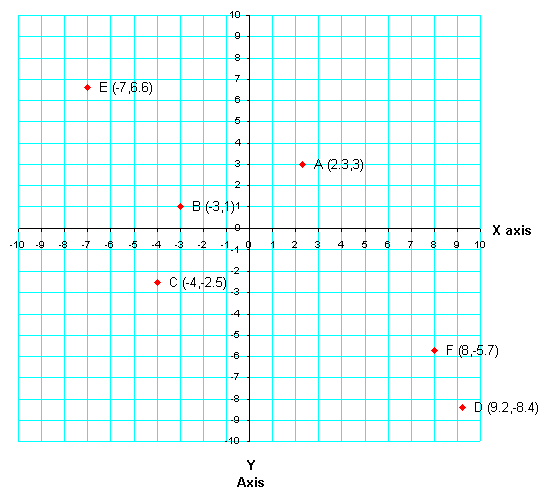
The origin below is where the x-axis and the y-axis meet. Point A has coordinates (2.3,3), since it is 2.3 units to the right and 3 units up from the origin. Point B has coordinates (-3,1), since it is 3 units to the left, and 1 unit up from the origin. Point C has coordinates (-4,-2.5), since it is 4 units to the left, and 2.5 units down from the origin. Point D has coordinates (9.2,-8.4); it is 9 units to the right, and 8.4 units down from the origin. Point E has coordinates (-7,6.6); it is 7 units to the left, and 6.6 units up from the origin. Point F has coordinates (8,-5.7); it is 8 units to the right, and 5.7 units down from the origin.

Comparing Positive and Negative Numbers

We can compare two different numbers by looking at their positions on the number line. For any two different places on the number line, the number on the right is greater than the number on the left. Note that every positive number is greater than any negative number.

Examples:

9.1 > 4, 6 > -9.3, -2 > -8, and 0 > -5.5
-2 < -13, -1 < -0.5, -7 < -5, and -10 < 0.1

Reciprocals of Negative Numbers

The reciprocal of a positive or negative fraction is obtained by switching its numerator and denominator, the sign of the new fraction remains the same. To find the reciprocal of a mixed number, first convert the mixed number to an improper fraction, then switch the numerator and denominator of the improper fraction. Notice that when you multiply negative fractions with their reciprocals, the product is always 1 (NOT -1).

Examples:

What is the reciprocal of -2/7? We just switch the numerator and denominator, and keep the same sign: -7/2.

What is the reciprocal of - 5 1/8? First, we convert to a negative improper fraction: -5 1/8 = - 41/8, then we switch the numerator and denominator, and keep the same sign: - 8/41.