Articles
Figures and Polygons
- Details
- Parent Category: Math League Website
- Published: 15 September 2008
Figures and polygons
Polygon
Regular polygon
Vertex
Triangle
Equilateral triangle
Isosceles triangle
Scalene triangle
Acute triangle
Obtuse triangle
Right triangle
Quadrilateral
Rectangle
Square
Parallelogram
Rhombus
Trapezoid
Pentagon
Hexagon
Heptagon
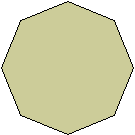
Octagon
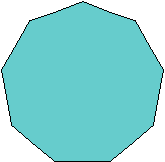
Nonagon
Decagon
Circle
Convex
Polygon
A polygon is a closed figure made by joining line segments, where each line segment intersects exactly two others.
Examples:
The following are examples of polygons:
The figure below is not a polygon, since it is not a closed figure:
The figure below is not a polygon, since it is not made of line segments:
The figure below is not a polygon, since its sides do not intersect in exactly two places each:
Regular Polygon
A regular polygon is a polygon whose sides are all the same length, and whose angles are all the same. The sum of the angles of a polygon with n sides, where n is 3 or more, is 180° × (n - 2) degrees.
Examples:
The following are examples of regular polygons:

Examples:
The following are not examples of regular polygons:
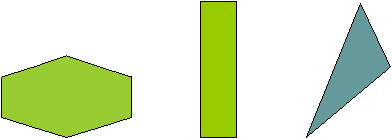
Vertex
1) The vertex of an angle is the point where the two rays that form the angle intersect.
2) The vertices of a polygon are the points where its sides intersect.
Triangle
A three-sided polygon. The sum of the angles of a triangle is 180 degrees.
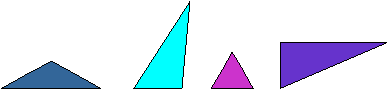
Examples:
Equilateral Triangle or Equiangular Triangle
A triangle having all three sides of equal length. The angles of an equilateral triangle all measure 60 degrees.
Examples:

Isosceles Triangle
A triangle having two sides of equal length.
Examples:
Scalene Triangle
A triangle having three sides of different lengths.
Examples:
Acute Triangle
A triangle having three acute angles.
Examples:

Obtuse Triangle
A triangle having an obtuse angle. One of the angles of the triangle measures more than 90 degrees.
Examples:

Right Triangle
A triangle having a right angle. One of the angles of the triangle measures 90 degrees. The side opposite the right angle is called the hypotenuse. The two sides that form the right angle are called the legs. A right triangle has the special property that the sum of the squares of the lengths of the legs equals the square of the length of the hypotenuse. This is known as the Pythagorean Theorem.
Examples:

Example:
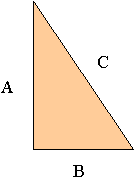
For the right triangle above, the lengths of the legs are A and B, and the hypotenuse has length C. Using the Pythagorean Theorem, we know that A2 + B2 = C2.
Example:
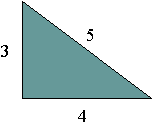
In the right triangle above, the hypotenuse has length 5, and we see that 32 + 42 = 52 according to the Pythagorean Theorem.
Quadrilateral
A four-sided polygon. The sum of the angles of a quadrilateral is 360 degrees.
Examples:
Rectangle
A four-sided polygon having all right angles. The sum of the angles of a rectangle is 360 degrees.
Examples:
Square
A four-sided polygon having equal-length sides meeting at right angles. The sum of the angles of a square is 360 degrees.
Examples:
Parallelogram
Parallelogram
A four-sided polygon with two pairs of parallel sides. The sum of the angles of a parallelogram is 360 degrees.
Examples:

Rhombus
A four-sided polygon having all four sides of equal length. The sum of the angles of a rhombus is 360 degrees.
Examples:
Trapezoid
A four-sided polygon having exactly one pair of parallel sides. The two sides that are parallel are called the bases of the trapezoid. The sum of the angles of a trapezoid is 360 degrees.
Examples:
Pentagon
A five-sided polygon. The sum of the angles of a pentagon is 540 degrees.
Example:
| A regular pentagon:
|
|
Hexagon
A six-sided polygon. The sum of the angles of a hexagon is 720 degrees.
Examples:
|
A regular hexagon: |
An irregular hexagon:
|
Heptagon
A seven-sided polygon. The sum of the angles of a heptagon is 900 degrees.
Examples:
| A regular heptagon:
|
An irregular heptagon:
|
Octagon
An eight-sided polygon. The sum of the angles of an octagon is 1080 degrees.
Examples:
| A regular octagon:
|
An irregular octagon:
|
Nonagon
A nine-sided polygon. The sum of the angles of a nonagon is 1260 degrees.
Examples:
| A regular nonagon:
|
An irregular nonagon:
|
Decagon
A ten-sided polygon. The sum of the angles of a decagon is 1440 degrees.
Examples:
|
A regular decagon:
|
An irregular decagon:
|
|
Circle
A circle is the collection of points in a plane that are all the same distance from a fixed point. The fixed point is called the center. A line segment joining the center to any point on the circle is called a radius.
Example:
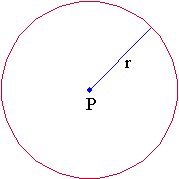
The blue line is the radius r, and the collection of red points is the circle.
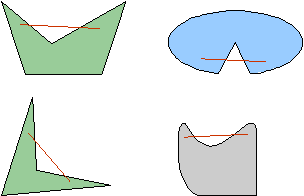
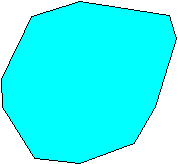
Convex
A figure is convex if every line segment drawn between any two points inside the figure lies entirely inside the figure. A figure that is not convex is called a concave figure.
Example:
The following figures are convex.
The following figures are concave. Note the red line segment drawn between two points inside the figure that also passes outside of the figure.